When dealing with absolute value graphs, students often make common mistakes in interpreting and sketching these graphs. Below is a breakdown of how to correctly understand and graph absolute value functions while avoiding common mistakes.
-
Understanding the Basic Absolute Value Graph
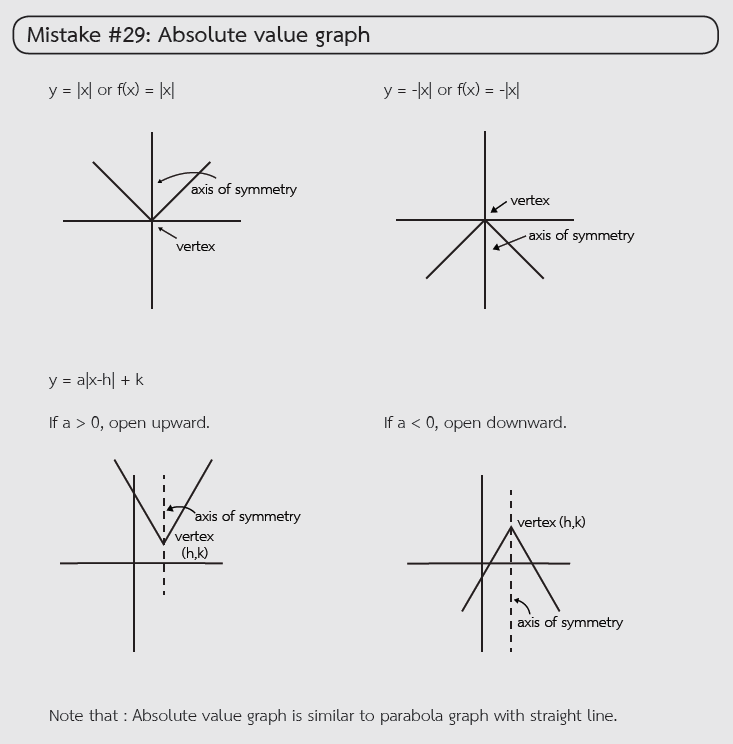
The graph of y = |x| or f(x) = |x| is a V-shaped graph with its vertex at the origin (0,0). The graph is symmetric around the vertical line called the axis of symmetry. This graph opens upwards, forming two straight lines. A common mistake is misinterpreting the slope or symmetry of the graph. -
Negative Absolute Value Graph
The graph of y = -|x| or f(x) = -|x| is an inverted V-shaped graph. This graph also has its vertex at the origin, but it opens downward. The axis of symmetry remains the same, but the graph now slopes downward on both sides. A common mistake here is misinterpreting the orientation of the graph and thinking it opens upwards. -
Graphing Transformed Absolute Value Functions
When the absolute value function is written in the form y = a|x – h| + k, the graph can be shifted and stretched depending on the values of a, h, and k. - If a > 0, the graph opens upward, with the vertex at (h, k).
- If a < 0, the graph opens downward, with the vertex still at (h, k).
A common mistake is misunderstanding how the value of a affects the direction the graph opens, or not recognizing that the vertex shifts based on h and k. -
Absolute Value Graph Similar to Parabola
An important note is that the absolute value graph is similar to the graph of a parabola, but with straight lines instead of curves. The graph of y = |x| and the graph of y = ax^2 both have a vertex and symmetry, but the parabola curves, while the absolute value graph has straight lines. A common mistake is confusing the two types of graphs and not recognizing the linearity of the absolute value graph.
By understanding these key points and common mistakes, you can more easily graph absolute value functions and correctly interpret their transformations. Always pay attention to the vertex, axis of symmetry, and the direction in which the graph opens based on the value of a.