Common Mistakes in Solving Absolute Inequalities and How to Solve Them Correctly

When working with absolute inequalities, students often encounter common mistakes that can lead to confusion and incorrect solutions. Understanding how to approach and solve these inequalities correctly is crucial. Let’s break down the types of absolute inequalities and how to avoid the typical errors that students make when solving them.

1. Understanding Absolute Value Less Than a Positive Number

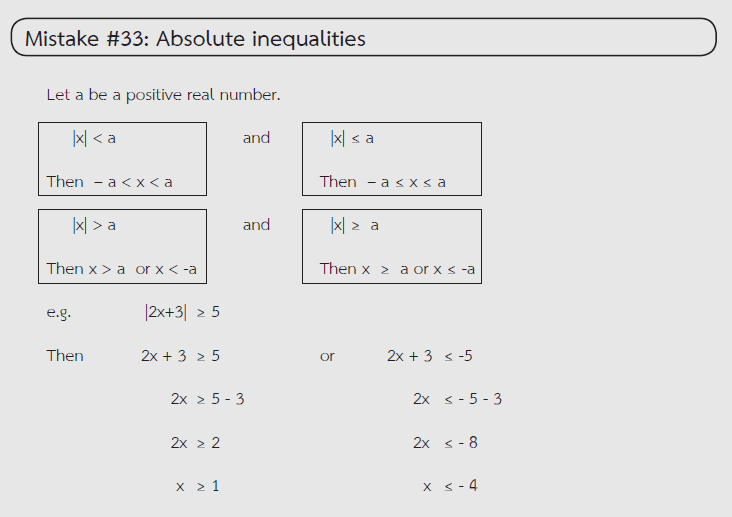
The first type of absolute inequality involves the absolute value of a number being less than a given positive value. In this case, the solution is a range that lies between the negative and positive value of the number. For example, if |x| < a, it means -a < x < a. A common mistake here is misinterpreting this inequality, leading students to either miss out on values or fail to properly solve the range of solutions.

2. Handling Absolute Value Greater Than a Positive Number

Next, when the absolute value of a number is greater than a positive value, the solution implies that the number is either greater than the positive value or less than the negative value. For example, if |x| > a, the solution is x > a or x < -a. This results in two separate conditions that must be solved independently. A frequent mistake is failing to break the inequality into these two parts, which leads to an incomplete or incorrect solution.

3. Inequalities with Less Than or Equal to (≤)

When the absolute value of a number is less than or equal to a given value, the solution includes all values between the negative and positive values, including the boundaries. For example, if |x| ≤ a, the solution is -a ≤ x ≤ a. Students sometimes make the mistake of not properly including the boundary values or misinterpreting the range, which can lead to an incorrect solution.

4. Example of Solving Absolute Inequalities

Consider the inequality |2x + 3| ≥ 5. This should be broken down into two separate cases:
Case 1: 2x + 3 ≥ 5
Case 2: 2x + 3 ≤ -5

Each case should be solved separately, and the final solution is the union of both results. A common mistake is either failing to solve one part of the inequality or solving it incorrectly, which results in an incomplete or incorrect answer.

5. Key Tips for Solving Absolute Inequalities

• Always break the inequality into separate cases: For inequalities involving greater than or less than, remember to split the problem into two conditions and solve them independently.
• Pay attention to boundary values: When the inequality is less than or equal to, ensure that you include the boundary values in your solution.
• Double-check your work: After solving each case, review the solutions to ensure both parts are considered correctly.

By understanding these common mistakes and knowing how to handle each situation properly, you can solve absolute inequalities confidently and accurately. Always remember to break down the inequality correctly and consider both possible solutions for inequalities involving absolute values.